Page 306 - 360.revista de Alta Velocidad - Nº 5

Balmaseda, Lucía. Gallego, Inmaculada. Sánchez-Cambronero, Santos. Rivas, Ana.

between the strength characteristics of the rail track and the dynamic coefficient .

2. Dynamic overloads calculation

2.1 Description of the numerical model

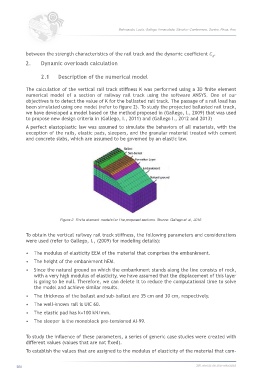
The calculation of the vertical rail track stiffness K was performed using a 3D finite element
numerical model of a section of railway rail track using the software ANSYS. One of our
objectives is to detect the value of K for the ballasted rail track. The passage of a rail load has
been simulated using one model (refer to figure 2). To study the projected ballasted rail track,
we have developed a model based on the method proposed in (Gallego, I., 2009) that was used
to propose new design criteria in (Gallego, I., 2011) and (Gallego I., 2012 and 2013)

A perfect elastoplastic law was assumed to simulate the behaviors of all materials, with the
exception of the rails, elastic pads, sleepers, and the granular material treated with cement
and concrete slabs, which are assumed to be governed by an elastic law.

Figure 2. Finite element models for the proposed sections. Source: Gallego et al, 2016.

To obtain the vertical railway rail track stiffness, the following parameters and considerations
were used (refer to Gallego, I., (2009) for modeling details):

• The modulus of elasticity EEM of the material that comprises the embankment.
• The height of the embankment hEM.
• Since the natural ground on which the embankment stands along the line consists of rock,
with a very high modulus of elasticity, we have assumed that the displacement of this layer
is going to be null. Therefore, we can delete it to reduce the computational time to solve
the model and achieve similar results.

• The thickness of the ballast and sub-ballast are 35 cm and 30 cm, respectively.
• The well-known rail is UIC 60.
• The elastic pad has k=100 kN/mm.

• The sleeper is the monoblock pre-tensioned AI-99.

To study the influence of these parameters, a series of generic case studies were created with
different values (values that are not fixed).

To establish the values that are assigned to the modulus of elasticity of the material that com-

304 360.revista de alta velocidad